#IDSky 🦋 Going to Week 3 - How Does It Look?

#IDSky Community, we’re onto week 3 and still going strong!

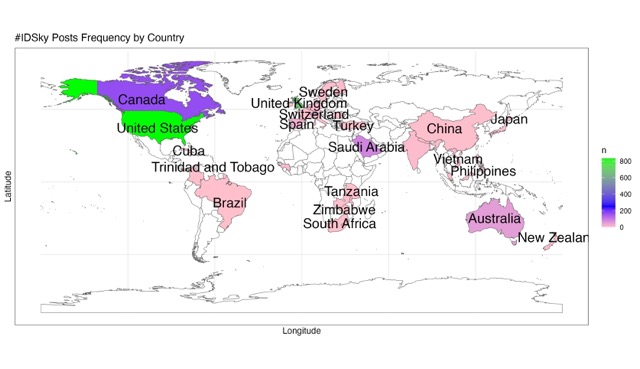
#IDSky 🦋 Week 2 - How Does It Look?

How’s #IDSky doing on week 2? Pretty great! We’re seeing steady posts worldwide, strong US-UK participation, and even outpacing Twitter metrics. The ID community isn’t just dropping by - they’re making BlueSky their new digital home.

🦋 Poll Tutorial For #IDSky

This is one way of creating an anonymous poll using poll.blue

A Step-by-step 🦋 Tutorial for #IDSky

BlueSky combines customizable filters, an ad-free experience, and free API access for data analysis. Here’s a simple guide to help you navigate the platform and connect with the #IDSky community.

Is #IDWeek Community Leaving X?

Not yet! After digging into both #IDWeek2023 and #IDWeek2024 tweets data, and crunching some numbers to compare user activity, it’s clear we haven’t lost our spark. Our ID community is still here, sharing knowledge and learning from each other’s experiences. Pretty cool to see how we’re keeping the conversation alive! Here’s hoping we can keep this momentum going and maybe even make it better!